
Painter: Jacopo De' Barbari 1460/70 – before 1516
Subject: Fra Luca Pacioli 1447–1517 (Geometer)
Painting: Portrait of Luca Pacioli 1495
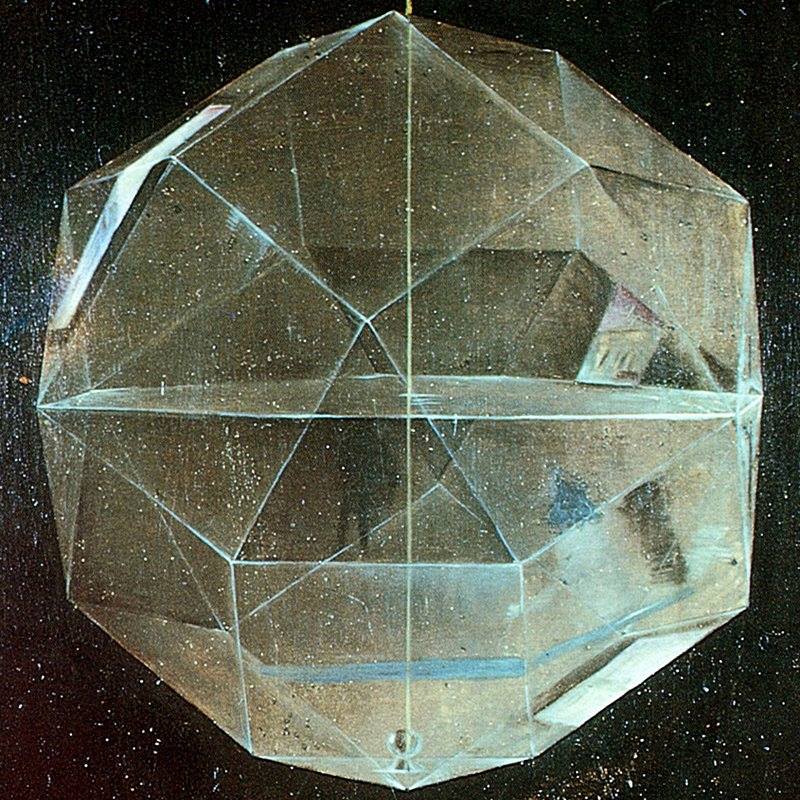
Hanging Object: Rhombicubotahedron more about
tennenbaum pacioli-divine-proportion (pdf)
https://www.scribd.com/document/244035060/tennenbaum-pacioli-divine-proportion-pdf
I never really thought of you as the magic math type.
neo-geo math
Newton's Prisms and His Experiments on the Spectrum
A. A. Mills
Notes and Records of the Royal Society of London
Vol. 36, No. 1 (Aug., 1981), pp. 13-36
http://www.jstor.org/stable/531655?seq=1#page_scan_tab_contents
A History of Color: The Evolution of Theories of Light and Color By Robert A. Crone
https://books.google.com/books?id=B-KtBAAAQBAJ&pg=PT102&lpg=PT102&dq=history+of+prism+grinding&source=bl&ots=xCB6_cUb5-&sig=5gXoG7HYPTWQ1cGEX03CcpYqK6E&hl=en&sa=X&ved=0ahUKEwjUia6nkcHZAhUotlkKHQTWDtQQ6AEIcjAM#v=onepage&q=history%20of%20prism%20grinding&f=false
If only that detail were the whole painting.
Steve I question "If only that detail were the whole painting"
It needs it all and it documents an amazing time in humanity, alchemy.....
Love it all.....love you all
there are other interesting elements to the painting, but its quite curious.
Enrico Gamba
Let's try to review the Double portrait of Luca Pacioli
Among the paintings belonging to the Renaissance in Urbino the one generally considered more mysterious and problematic is undoubtedly the Flagellation of Piero della Francesca. There is however another painting, always of Urbino origin, equally mysterious and problematic, it is the so-called Double portrait of the Venetian painter Iacopo de 'Barbari. The Double portrait is a painting of the best known and reproduced, I would say literally looted for advertising purposes, and yet has not found a public interest comparable to the Flagellation of Piero. It has indeed been the subject of specialist studies, which never however came to books such as those of Ginzburg, Calvesi, King, and Ronchey. I also have the impression, probably wrong, that we are resigned to accepting the riddles, so to speak, losing as the hope of understanding something more.
The problems that the painting proposes are different, starting from the attribution to Iacopo de 'Barbari certified by the cartouche, which the analyzes carried out at the Urbino exhibition in 1992 confirmed.
Other problems concern the two characters depicted. If the identity of Friar Luca Pacioli is out of the question, it is not the same as the second character traditionally recognized as the Duke Urbinate Guidubaldo da Montefeltro. The doubts also arose after a confrontation with a painting of clear Leonardesque influence preserved in London in the National Gallery, which portrays this Francesco di Bartolomeo Archinto, very similar to the character of the Double portrait. I think the identification of the second character is an open problem, to give an example we do not know where and on what occasion Iacopo de 'Barbari and Guidubaldo met.
Occupying myself with the history of science, the painting interests me above all from this point of view because it presents a series of truly unique exceptions. It is the first pictorial representation of a mathematician alive and well in action, surrounded by tools of the trade, immersed in his field of action. This is quite different from the allegorical representation of the sciences or the "auctoritates" as was the case for the portraits of Euclid and Ptolemy in the study of Duke Federico.
The painting was performed under the personal direction of Pacioli. The details are desired, clear, they can not have been done by the painter on their own initiative, you want to carry out a precise speech. It is about the representation of a concrete situation, not of generic or formal references.
Why waste so much care in so many details? I think that one of the aims of the painting is to launch a challenge: with the left hand Pacioli indicates a well defined and identifiable theorem of Euclid's Elements, with a wand in the right hand pointing on the slate an unfinished geometric figure, there is a triangle inscribed in a circumference and there is a segment that floats, which does not end up anywhere. Pacioli challenges us to solve a problem, he wants us to complete the figure, he wants us to say what it is.
In addition to the geometric figure, on the slate we find below the sum 478 + 935 + 621 = 2034. What interpretation to give these numbers? Are they randomly chosen? Knowing Pacioli it is difficult to be so.
The copy of the Elements placed on the table corresponds to the first printed edition, Venice 1482, the text is open to a specific page, the one with the proposition VIII of the book XIII: the square of the side of the equilateral triangle inscribed in a circumference is three times the square of the semidiameter of the circumference. Below the proposition IX: the golden section of the semidiameter of a circumference is the side of the regular decagon inscribed in it. The Pacioli index is aimed at the beginning of proposition VIII. It seems that this is the proposition in question. What does that have to do with the figure left incomplete on the blackboard, and has anything to do with the four numbers?
One possible answer may be the following. Proposition VIII, book XIII - the square of the side of the triangle is three times the square of the semidiameter - it relates the semidiameter and the side of the inscribed triangle. Continuing along the same line one can think of a relationship between the semidiameter and the side of the square inscribed in the same circumference. In this case the incomplete segment would be the side of the inscribed square, which would be completed at point X. The relationship between the sides of the triangle and the square inscribed in the same circumference is obtained: if the square of the side of the square is equal to double the square of the semidiameter, then the relation that the square of the side of the square is 2/3 of the square of the side of the equilateral triangle is worth.
The hypothesis can be indirectly confirmed by the numerical calculation: 478 + 935 + 621 = 2034. At first glance these are not particular numbers,numbers friends, perfect, etc., you immediately notice that in the three numbers added there are no repeated figures. The only one we say harmony that I found is the following: if 621 is the perimeter of an equilateral triangle of side 207, then 478 is the perimeter of the square built on the radius of the circumscribed circumference. Or in proposition VIII, in the book XIII Euclide surveyor relates the surfaces, while Pacioli which is also arithmetic and algebraic, relates the perimeters according to the use of the environment of practical mathematics, or abkistic mathematics, of always dealing with numerical cases . Even the three segments drawn in the upper corner of the blackboard gain a sense, representing the three dimensions in play: the semidiameter, the side of the square and the side of the equilateral triangle inscribed.A last clue in favor of this hypothesis is that the rhombicubottahedron hanging is made up of squares and equilateral triangles. But, I repeat, hypotheses are all to deny, my intent is only to arouse new interest in this work. (google translate) http://www.hevelius.it/webzine/leggi.php?codice=242 via:
Imagine Math 3: Between Culture and Mathematics
edited by Michele Emmer (google books)
Shaping Space: Exploring Polyhedra in Nature, Art, and the Geometrical ... edited by Marjorie Senechal
https://books.google.com/books?id=kZtCAAAAQBAJ&pg=PA57&dq=Fra+Luca+Pacioli+Rhombicuboctahedron+glass+model&hl=en&sa=X&ved=0ahUKEwj577WZ4NrZAhUnTt8KHR5sApgQ6AEINDAC#v=onepage&q=Fra%20Luca%20Pacioli%20Rhombicuboctahedron%20glass%20model&f=false
|
- bill 3-06-2018 11:15 am
- bill 3-06-2018 11:16 am [add a comment]
Painter: Jacopo De' Barbari 1460/70 – before 1516
Subject: Fra Luca Pacioli 1447–1517 (Geometer)
Painting: Portrait of Luca Pacioli 1495
Hanging Object: Rhombicubotahedron more about
- bill 3-06-2018 11:23 am [add a comment]
tennenbaum pacioli-divine-proportion (pdf)
https://www.scribd.com/document/244035060/tennenbaum-pacioli-divine-proportion-pdf
- bill 3-06-2018 11:56 am [add a comment]
I never really thought of you as the magic math type.
- alex 3-06-2018 12:27 pm [add a comment]
neo-geo math
- bill 3-06-2018 4:37 pm [add a comment]
Newton's Prisms and His Experiments on the Spectrum
A. A. Mills
Notes and Records of the Royal Society of London
Vol. 36, No. 1 (Aug., 1981), pp. 13-36
http://www.jstor.org/stable/531655?seq=1#page_scan_tab_contents
- bill 3-06-2018 7:07 pm [add a comment]
A History of Color: The Evolution of Theories of Light and Color By Robert A. Crone
https://books.google.com/books?id=B-KtBAAAQBAJ&pg=PT102&lpg=PT102&dq=history+of+prism+grinding&source=bl&ots=xCB6_cUb5-&sig=5gXoG7HYPTWQ1cGEX03CcpYqK6E&hl=en&sa=X&ved=0ahUKEwjUia6nkcHZAhUotlkKHQTWDtQQ6AEIcjAM#v=onepage&q=history%20of%20prism%20grinding&f=false
- bill 3-06-2018 7:51 pm [add a comment]
If only that detail were the whole painting.
- steve 3-07-2018 10:30 am [add a comment]
Steve I question "If only that detail were the whole painting"
It needs it all and it documents an amazing time in humanity, alchemy.....
Love it all.....love you all
- Skinny 3-09-2018 9:26 pm [add a comment]
there are other interesting elements to the painting, but its quite curious.
- bill 3-07-2018 10:37 am [add a comment]
Enrico Gamba
Let's try to review the Double portrait of Luca Pacioli
Among the paintings belonging to the Renaissance in Urbino the one generally considered more mysterious and problematic is undoubtedly the Flagellation of Piero della Francesca. There is however another painting, always of Urbino origin, equally mysterious and problematic, it is the so-called Double portrait of the Venetian painter Iacopo de 'Barbari. The Double portrait is a painting of the best known and reproduced, I would say literally looted for advertising purposes, and yet has not found a public interest comparable to the Flagellation of Piero. It has indeed been the subject of specialist studies, which never however came to books such as those of Ginzburg, Calvesi, King, and Ronchey. I also have the impression, probably wrong, that we are resigned to accepting the riddles, so to speak, losing as the hope of understanding something more.
The problems that the painting proposes are different, starting from the attribution to Iacopo de 'Barbari certified by the cartouche, which the analyzes carried out at the Urbino exhibition in 1992 confirmed.
Other problems concern the two characters depicted. If the identity of Friar Luca Pacioli is out of the question, it is not the same as the second character traditionally recognized as the Duke Urbinate Guidubaldo da Montefeltro. The doubts also arose after a confrontation with a painting of clear Leonardesque influence preserved in London in the National Gallery, which portrays this Francesco di Bartolomeo Archinto, very similar to the character of the Double portrait. I think the identification of the second character is an open problem, to give an example we do not know where and on what occasion Iacopo de 'Barbari and Guidubaldo met.
Occupying myself with the history of science, the painting interests me above all from this point of view because it presents a series of truly unique exceptions. It is the first pictorial representation of a mathematician alive and well in action, surrounded by tools of the trade, immersed in his field of action. This is quite different from the allegorical representation of the sciences or the "auctoritates" as was the case for the portraits of Euclid and Ptolemy in the study of Duke Federico.
The painting was performed under the personal direction of Pacioli. The details are desired, clear, they can not have been done by the painter on their own initiative, you want to carry out a precise speech. It is about the representation of a concrete situation, not of generic or formal references.
Why waste so much care in so many details? I think that one of the aims of the painting is to launch a challenge: with the left hand Pacioli indicates a well defined and identifiable theorem of Euclid's Elements, with a wand in the right hand pointing on the slate an unfinished geometric figure, there is a triangle inscribed in a circumference and there is a segment that floats, which does not end up anywhere. Pacioli challenges us to solve a problem, he wants us to complete the figure, he wants us to say what it is.
In addition to the geometric figure, on the slate we find below the sum 478 + 935 + 621 = 2034. What interpretation to give these numbers? Are they randomly chosen? Knowing Pacioli it is difficult to be so.
The copy of the Elements placed on the table corresponds to the first printed edition, Venice 1482, the text is open to a specific page, the one with the proposition VIII of the book XIII: the square of the side of the equilateral triangle inscribed in a circumference is three times the square of the semidiameter of the circumference. Below the proposition IX: the golden section of the semidiameter of a circumference is the side of the regular decagon inscribed in it. The Pacioli index is aimed at the beginning of proposition VIII. It seems that this is the proposition in question. What does that have to do with the figure left incomplete on the blackboard, and has anything to do with the four numbers?
One possible answer may be the following. Proposition VIII, book XIII - the square of the side of the triangle is three times the square of the semidiameter - it relates the semidiameter and the side of the inscribed triangle. Continuing along the same line one can think of a relationship between the semidiameter and the side of the square inscribed in the same circumference. In this case the incomplete segment would be the side of the inscribed square, which would be completed at point X. The relationship between the sides of the triangle and the square inscribed in the same circumference is obtained: if the square of the side of the square is equal to double the square of the semidiameter, then the relation that the square of the side of the square is 2/3 of the square of the side of the equilateral triangle is worth.
The hypothesis can be indirectly confirmed by the numerical calculation: 478 + 935 + 621 = 2034. At first glance these are not particular numbers,numbers friends, perfect, etc., you immediately notice that in the three numbers added there are no repeated figures. The only one we say harmony that I found is the following: if 621 is the perimeter of an equilateral triangle of side 207, then 478 is the perimeter of the square built on the radius of the circumscribed circumference. Or in proposition VIII, in the book XIII Euclide surveyor relates the surfaces, while Pacioli which is also arithmetic and algebraic, relates the perimeters according to the use of the environment of practical mathematics, or abkistic mathematics, of always dealing with numerical cases . Even the three segments drawn in the upper corner of the blackboard gain a sense, representing the three dimensions in play: the semidiameter, the side of the square and the side of the equilateral triangle inscribed.A last clue in favor of this hypothesis is that the rhombicubottahedron hanging is made up of squares and equilateral triangles. But, I repeat, hypotheses are all to deny, my intent is only to arouse new interest in this work. (google translate) http://www.hevelius.it/webzine/leggi.php?codice=242 via:
Imagine Math 3: Between Culture and Mathematics
edited by Michele Emmer (google books)
- bill 3-07-2018 12:29 pm [add a comment]
Shaping Space: Exploring Polyhedra in Nature, Art, and the Geometrical ... edited by Marjorie Senechal
https://books.google.com/books?id=kZtCAAAAQBAJ&pg=PA57&dq=Fra+Luca+Pacioli+Rhombicuboctahedron+glass+model&hl=en&sa=X&ved=0ahUKEwj577WZ4NrZAhUnTt8KHR5sApgQ6AEINDAC#v=onepage&q=Fra%20Luca%20Pacioli%20Rhombicuboctahedron%20glass%20model&f=false
- bill 3-07-2018 12:43 pm [add a comment]